Hello Roger

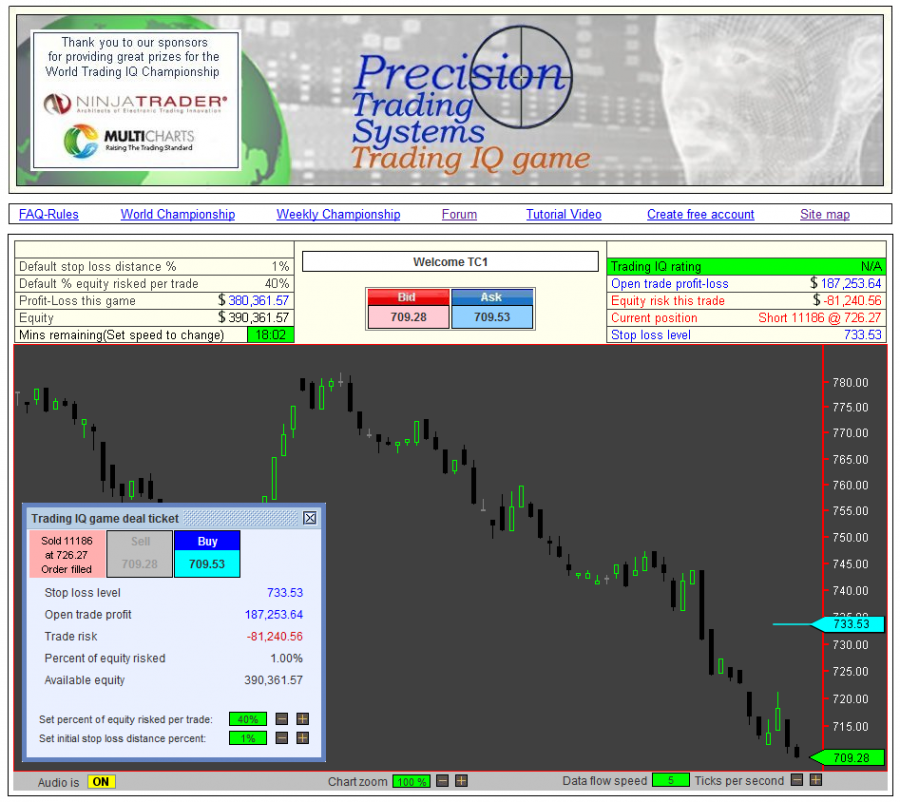
This evening I tested playing the game on your site with a 1% stop loss and 35% risk per each trade, then I slowed the game down to 2 ticks per second.

I ran my $10,000 capital up to $995,679 at one point and ended up with $157,543 equity from just one game!!

Before I did not notice the stop loss and risk percentage were adjustable and wow what a massive difference they make.

Looks like I might have a chance to be the world champion for this year.

Please see the screenshot to prove I did it.

Congratulations on your high score and learning the effects of risk management. One of the main purposes of this game as well as teaching people how to get a feel for price action was to educate users on how drastic the results will vary when changing risk per trade and stop distances as a combination. Using 40% risk per trade with a 1% stop allows huge leverage thus. $10,000 starting capital x 40% = $4000 risk Share price of 750 and 1% stop loss = 7.5 points risked ( assuming it does not gap through the stop ) IMPORTANT POINT ! If a non leveraged 100% stop loss was used then it would buy $4000 worth of shares at $750 each making 5.33 shares rounded to 5. As it is just 1% stop loss then you end up with 100 times more shares 533 x $750 each = $400,000 trade value or 40 times gearing. So in a nutshell, risk per trade set as constant and stop changed from 20% to 1% makes a 20 times increase in deal size. Great things can happen to your account with such high risk but this is dampened by the risk of a price gapping through your stop loss and a mere 2.5 gap below your stop is a total wipe out of the account. Also a string of three consecutive losing trades would reduce a $10,000 account thus. 1st 40% loss = $6000 left 2nd 40% loss = $3600 left 3rd 40% loss = $2160 left You might consider this analogy to set your risk principles in proportion. QUESTION A man sells his home for $500,000 and goes to the casino and decides to put it all on black for three consecutive spins If 100 men try this, based on statistical probabilities how many will lose everything? Also based on statistical probabilities how many men will hit 3 blacks in a row to win $500,000 followed by $1million ( 2nd spin ) and $2million on the third spin making their total equity $4million? To compute this our roulette wheel has one slot with a green 0 ( no payout for a bet on black or red ) and 18 black slots and 18 red slots. Hence there are 37 slots total and 18 black slots. Therefore there is a 48.64% chance of the ball landing on black on any of the spins but if the first or second spin lose then no more bets are made as there is no money left. Work it out yourself before scrolling down to the answer After the first spin we are left with 48.64 men with $1million each and 51.36 men broke After the 2nd spin we are left with 23.66 men with $2 million each and 76.33 men broke After the 3rd spin we are left with 11.50 men who go home rich with $4 million each and 88.50 men who go home broke. So in the case of roulette it really is a case of the more you play the more you lose, and if you really must go crazy the maths prove that just doing it once is the best chance of winning. The problem is the agony of choice between if to bet on red or black.